Random Exercise #5: The homotopy fibre of CP^1 ↪ CP^∞ and a Serre Spectral Sequence

2 May 2017 by RicPed Leave a comment

[It is really annoying that the title cannot contain any tex code:( ]

Hi all, sorry for not having written much in the blog lately, i was kind of busy. First of all, I’m happy to announce that next I’ll be a graduate student at UT in Austin. I’m so happy and I’m looking forward to start working there. Ok, back to business, on today’s random exercise I will deal with a problem I encountered today. Since I found it funny and interesting I will write it down here. We are asked to study the homotopy fibre of the map

$\mathbb{C}P^1 \hookrightarrow \mathbb{C}P^{\infty}$ .

Let us call such homotopy fibre $F$ . First step is to compute its homotopy groups. A quick application of the l.e.s. of a fibration together with the fact that the inclusion induces an isomorphism in degree $0,1,2$ gives that:

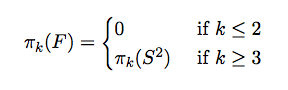
Screen Shot 2017-05-02 at 21.54.44

Now we turn our attention to the integral cohomology of $F$ via a spectral sequence argument. God, I love spectral sequences 🙂

So the second page of the Serre Spectral sequence for the fibration $F \to \mathbb{C}P^1 \to \mathbb{C}P^{\infty}$ looks like this

Screen Shot 2017-05-02 at 22.11.21.png

where in the upper rows I used the canonical isomorphism:

Screen Shot 2017-05-02 at 22.17.51

which can be obtained in several ways, UCT mainly. This is to stress the fact that we are going to make heavy use of the multiplicative structure of this SSeq.

Claim 1: The differential $d_{p,q}^k \colon E^{p,q}_k \to E^{p+k,q-k+1}_k$ is uniquely determined by the differential $d_{0,q}^k \colon E^{0,q}_k \to E^{k,q-k+1}_k$

Proof. This is just an application of the multiplicativity of the SSeq together with the ring structure of the cohomology ring of $\mathbb{C}P^{\infty}$ . In fact we have that $d^k_{2p,q}(\lambda \cdot x^p )=d^k_{2p,q}(\lambda\cdot 1 \smile 1\cdot x^p ) = d^k_{0,q}(\lambda)\smile x^p \pm \lambda \smile d^k_{p,0}(x^p)$

but $d^k_{p,0}=0$ since it is a first quadrant spectral sequence. Therefore we see that

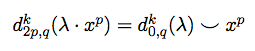
Screen Shot 2017-05-02 at 23.11.57

Proving the claim.

Remark: Since $H^{p+q}(S^2)=0$ for $p+q\geq 3$ , it should be clear to the reader that on the stable page the third, fourth, fifth and so on diagonals have to be trivial, i.e. $E_{\infty}^{p,q}=0$ whenever $p+q\geq 3$. It’s should be easy to understand why, if this is weren’t true, then we would obtain a non-trivial $H^{p+q}(S^2)$ .

Claim 2: $d^4 \colon H^3(F) \xrightarrow{\cong} H^0(F)$

Proof. before proving it, it’s clear that we made a little abuse of notation, what we really mean is that $d^4_{0,3} \colon E_4^{0,3}\to E_4^{4,0}$ is an isomorphism. Let’s have a look at the third diagonal: the only possibly non zero object is $E_{\infty}^{0,3}$ . In order to ensure its triviality the only possibility (check it!) is that $d_{0,3}^4$ is injective. Now look at the fourth diagonal. In order to ensure the triviality of $E_{4,0}^{\infty}$ the only possibility is that $latex d_{0,3}^4$ is surjective. In fact it’s the only differential hitting it.

Claim 3: $H^4(F)=0$ .

Proof. Looking at the fourth diagonal, it’s clear (by the same reasoning as above) that in order to ensure $E_{\infty}^{0,4}=0$ , $d_{0,4}^2$ has to be injective (again, it’s the only possibly non-trivial differential hitting/starting from position $(0,4)$ ). Since differential are, well, differential ( $d^k\circ d^k=0$ ) together with Claim 1 we have that $d_{p,4}^2=0$ for all $p$ . In particular being an injection it must be that $H^4(F)=0$ as claimed.

Claim 4: $H^q(F)=0$ for $q\geq 4$

Proof. The reasoning now is the same as above, and is done inductively. I will show in detail the step for $q=5$ .

Screen Shot 2017-05-02 at 22.59.17.png

In the picture you can find that the only possible way to kill $E_{\infty}^{0,5}$ is by means of $d^6_{0,5}$ (the others, as shown, land in zeroes). Unfortunately, $E_6^{6,0}=0$ by Claim 2 + Remark. So the only possibility is that $H^5(F)=0$ as claimed. The other cases are done similarly.

We can conclude that the homotopy fibre $F$ of $\mathbb{C}P^1 \hookrightarrow \mathbb{C}P^{\infty}$ is a Moore Space of type $(\mathbb{Z},3)$ .

Update #1: I realised we can be more precise with the identification of the fibre: First of all, the map we are interested in, via cellular approximation can be homotope to the map $i\colon S^2 \hookrightarrow \mathbb{C}P^2$ . Then we use the (weak) homotopy equivalence between

$F \cong hofib(i) \simeq \Omega hocofib(i)$

Since $i$ is clearly a cofibration (CW inclusion), its homotopy cofibre is simply its cone, but the cone of $latex S^2 \hookrightarrow \mathbb{C}P^2$ is homotopy equivalent to $S^4$ , since we are simply “killing” the cell in dimension $2$ , leaving just the $0$ th and $4$ th cell. Now it’s easy to see that we have an homotopy equivalence $\Omega S^4 \simeq S^3$ (by Whitehead theorem applied to the map $S^3 \to \Omega \Sigma S^3=\Omega S^4$ given by the usual adjunction). Since the homotopy fibre is defined up to homotopy, we can take as a model for it the $3$ -sphere (which is a Moore Space of type $(\mathbb{Z},3)$ clearly )

Update #2: Actually I realised it’s way easier proving that $F \simeq S^3$ . Recall we proved that $\pi_1(F)=\pi_2(F)=0$ and that $\pi_3(F)=\mathbb{Z}$ . Take a representative of the generator of this group and call it $j \colon S^3 \to F$ . With this map (it’s important to have an actual map!) one can consider $S^3 \subseteq F$ , and it’s really easy to realise that $\pi_1(F,S^3)=\pi_2(F,S^3)=0$ via the long exact sequence. Since we chose $j$ cleverly, it turns out that $\pi_3(F,S^3)=0$ (again, by the l.e.s.). An application of the l.e.s. in homology for the pair $(F,S^3)$ together with Hurewicz gives that $H_*(F,S^3)=0$ . Since $S^3$ is simply connected, by the relative Whitehead Theorem ([FoFu], Lecture 14.5 page 183) we have that $\pi_*(F,S^3)=0$ for all $k$ and $j$ is therefore a weak homotopy equivalence. Since the homotopy fiber is a CW complex, we have that $j$ is even an homotopy equivalence, and therefore we have the claim.